Novel tensor network methods for correlated quantum systems
The main research interests of the Focus Group were in the mathematical aspects of novel tensor network state (TNS) methods and their application to strongly correlated quantum many-body systems. These methods can be used to simulate and study magnetic properties in solid states, exotic quantum phases, complex molecular clusters, ultracold atomic systems, and nuclear structures on high-performance computing infrastructures.

Focus Group: Novel Tensor Network Methods for Strongly Correlated Quantum Systems
Prof. Örs Legeza (Wigner Research Centre for Physics), Alumnus Hans Fischer Senior Fellow | Elizabeth Adomako Boamah (TUM), Doctoral Candidate | Host: Prof. Gero Friesecke (TUM)
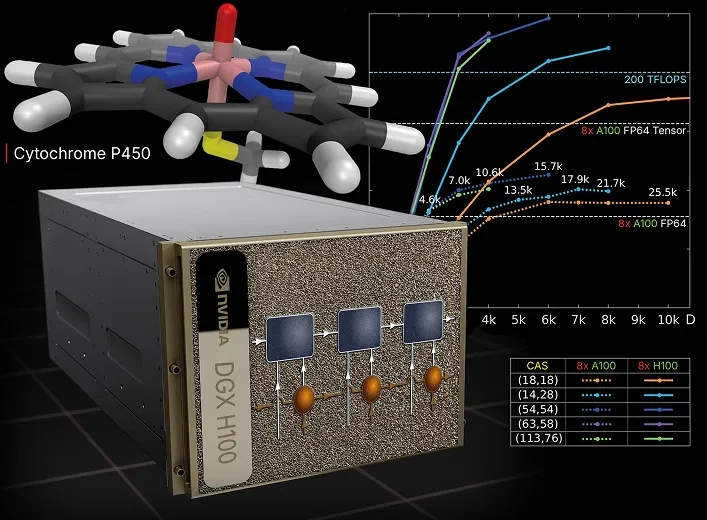
For the method development, we combined established methods for simple networks with concepts from quantum information theory, computational mathematics, and high-performance computing to push the current frontier of moderate system sizes to much larger and more complex systems. By performing, for example, electronic structure calculations via our state-of-the-art TNS code, we achieved a quarter petaflops performance on a single DGXH100 GPU accelerated hardware platform for the active compounds of the FeMoco and cytochrome P450 (CYP) enzymes with complete active space (CAS) sizes of up to 113 electrons in 76 orbitals [CAS(113, 76)] and 63 electrons in 58 orbitals [CAS(63, 58)], respectively. This incredible computational power has the potential to pave the way for simulation of challenging multi-reference problems in chemistry or highly correlated materials science, i.e., to perform largescale, high-accuracy ab initio computations routinely on a daily basis for a broad range of disciplines.
Altogether, during the three-year time period of the Fellowship, our research work resulted in 27 publications in high-quality journals including Phys. Rev. Lett, Phys. Rev. B, Phys. Rev. Research, Phys. Let. B, J. Chem. Theory Comput., J. Math. Chem., J. Chem. Phys, and npj Computational Materials among many others, each indicating the support provided by the TUM-IAS. Fundamental results have been achieved in condensed matter physics focusing on new exotic quantum phases of matter, such as Wigner necklace in carbon nanotubes, Z4 parafermions, hadron formation in repulsive Hubbard Models, and formation of chemically stable spin qubits in hexagonal boron nitride. Extensions to time-dependent phenomena and dissipating quantum systems have also been part of our research. We have also introduced novel methods to locate and characterize quantum phase transitions, focusing on the most difficult infinite order Kosterlizt-Thouless phase transition and on Mott-Hubbard transition in model systems without upklapp scatterings.
In nuclear structure theory, we have opened a new research direction via tensor network state methods by studying neutron- and proton-rich heavy isotopes, also bringing into the field concepts of quantum information theory and entanglement-based analysis tools. From a method development point of view, we have combined the in-medium similarity renormalization group (ISMRG) approach with the density matrix renormalization group (DMRG) method and demonstrated the very stable and robust convergence of the new method (VS-DMRG) on hard problems that are beyond the scope of conventional algorithms.
Figure 1
In quantum chemistry, we have studied convergence of a fully correlated wave function in the infinite basis limit via a rigorous mathematical framework based on matrix product state representation. We have also introduced novel methods by combining the density matrix renormalization group (DMRG) method with the concept of restricted active space (RAS) and have shown that the new method has the potential to outperform conventional methods such as coupled cluster (CC) and multi-reference configuration interaction (MRCI). In addition, on the basis of rigorous scaling analysis, we have developed a new extrapolation approach (DMRG-RAS-X) to predict full-CI (exact) energies of large chemical systems. We studied several strongly correlated (multi-reference) molecular systems, also from the point of view of quantum information theory.
By combining our complementary expertise, we aimed to find an optimal representation of a quantum many-body wave function, i.e., a parametrization with the minimum number of parameters for a given error margin, which is a task of the utmost importance in modern quantum physics and chemistry. To this end, we have developed novel algorithmic solutions to further boost the performance of tensor network state methods based on global fermionic mode optimization. This new approach has the potential to revolutionize simulations on two and higher dimensional quantum lattice models, demonstrated via large-scale numerical simulations, especialespecially when massively parallel computations are performed on high-performance computing infrastructures.
We have also developed a new code base to carry out proof-of-principle calculations to study the effect of tensor network topologies when one or several loops are introduced. Various interpolations between limiting cases have been determined, and our preliminary results indicate lower numerical errors for a broad parameter regime. This project also formed the basis of the HFSF PhD program, and the related doctoral degree of our PhD candidate is anticipated in the near future.
We have further developed our software package exploiting the enormous computational power offered by high-performance computing centers, and we initiated various collaborations with industrial partners, including NVIDIA, AMD, SandboxAQ (Google startup), Furukawa Electronic Institute of Technology, RiverLane Ltd, FACCTS GMBH (spin-off of the Max Planck Society). Moreover, our DMRG code has been used by some 30 research groups worldwide in condensed matter and nuclear physics and in quantum chemistry.
We have organized three international workshops. The one at TUM-IAS took place in April 2024, bringing together condensed matter physicists, mathematicians, and theoretical chemists to continue the exploration of tensor network state methods and to stimulate further developments. Numerous prominent scientists from the field were invited as speakers for the workshop, and young researchers also attended and contributed to the workshop with talks and posters. The focus of the workshop was on innovative ideas for moving beyond the current limits of quantum many-body simulations despite the major challenges of high dimensionality and accuracy.
During the past three-year time period, we have presented our research results via close to 45 international conferences, workshops, and seminar talks. We have had active collaborations with some ten universities and research institutes in Germany. Among others, follow-up collaborations have also been established recently by CERN, the Parmenides Foundation, and Argonne National Laboratory.
Selected publications
- Menczer, A., van Damme, M., Rask, A., Huntington, L., Hammond J., Xantheas, S. S., Ganahl, M. & Legeza, Ö. Parallel implementation of the Density Matrix Renormalization Group method achieving a quarter petaFLOPS performance on a single DGX-H100 GPU node, J. Chem. Theory Comput. 20, 19, 8397–8404 (2024).
- Friesecke, G., Werner, M. A., Kapás, K., Menczer, A. & Legeza, Ö. Global fermionic mode optimization via swap gates, arXiv:2406.03449 (2024).
- Menczer A., Kapás, K, Werner, M. A. & Legeza, Ö. Two dimensional quantum lattice models via mode optimized hybrid CPU-GPU density matrix renormalization group method, Phys. Rev. B 109, 195148 (2024).
- Friesecke G., Barcza G. & Legeza Ö. Predicting the FCI energy of large systems to chemical accuracy from restricted active space density matrix renormalization group calculations, J. Chem. Theory Comput., 20, 1, 87–102 (2024).
- Friesecke, G. Graswald, B. R., & Legeza, Ö. Exact matrix product state representation and convergence of a fully correlated electronic wavefunction in the infinite-basis limit. Physical Review B 105, 16, 165144 (2022).